Ordered exponential

The ordered exponential (also called the path-ordered exponential) is a mathematical object, defined in non-commutative algebras, which is equivalent to the exponential function of the integral in the commutative algebras. Therefore it is a function, defined by means of a function from real numbers to a real or complex associative algebra. In practice the values lie in matrix and operator algebras.

For the element A(t) from the algebra 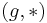$(g,*)$ (set g with the non-commutative product *), where t is the "time parameter", the ordered exponential $OE[A](t):\equiv \left(e^{\int_0^t dt' A(t')}\right)_%2B$ of A can be defined via one of several equivalent approaches:

As the limit of the ordered product of the infinitesimal exponentials:

$OE[A](t) = \lim_{N \rightarrow \infty} \left\{ e^{\epsilon A(t_N)}*e^{\epsilon A(t_{N-1})}* \cdots *e^{\epsilon A(t_1)}*e^{\epsilon A(t_0)}\right\}$

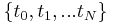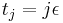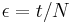where the time moments $\{t_0, t_1, ... t_N\}$ are defined as $t_j = j\epsilon$ for $j=0, ... N$ , and $\epsilon = t/N$ .

Via the initial value problem, where the OE[A](t) is the unique solution of the system of equations:

$\frac{\partial OE[A](t)}{\partial t} = A(t) * OE[A](t),$

$OE[A](0) = 1.$

Via an integral equation:

$OE[A](t) = 1 %2B \int_0^t dt' A(t') * OE[A](t').$

Via Taylor series expansion:

$OE[A](t) = 1 %2B \int_0^t dt_1 A(t_1) %2B \int_0^t dt_1 \int_0^{t_1} dt_2 A(t_1)*A(t_2)$

$%2B \int_0^t dt_1 \int_0^{t_1} dt_2 \int_0^{t_2} dt_3 A(t_1)*A(t_2)*A(t_3) %2B \cdots$

Ordered exponential

See also